Dirakova delta funkcija

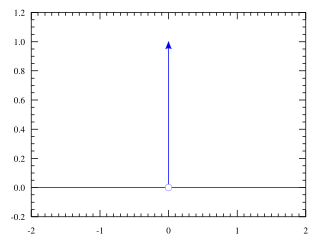
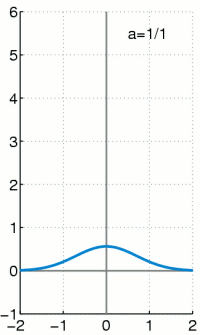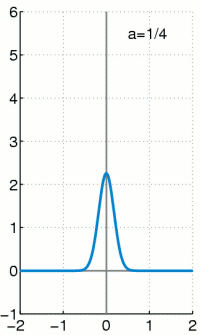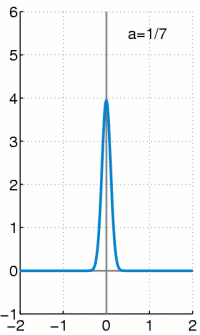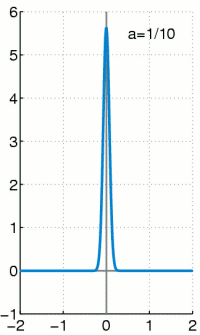
Šematski prikaz Direkove delta funkcije linijom na čijem vrhu je strelica. Visina strelice se obično koristi za specificiranje vrednosti multiplikativne konstante, koja daje površinu ispod oblasti ispod funkcije. Druga vrsta zapisivanja je da se napiše površina pored strelice.
Dirakova delta funkcija je granična vrednost svih normalnih raspodela sa maksimumom u nuli. δ a ( x ) = 1 a π e − x 2 a 2 {\displaystyle \delta _{a}(x)={\frac {1}{a{\sqrt {\pi }}}}\mathrm {e} ^{\frac {-x^{2}}{a^{2}}}} kada a → 0

Dirakova (delta) funkcija ili δ funkcija se opisuje kao funkcija u realnoj ravni, čija je vrednost u svim tačkama 0, osim u tački 0 kada iznosi beskonačno mnogo, definisana tako da je njen integral po celoj oblasti definsanosti 1.

δ funkciju je formulisao teoretski fizičar Pol Dirak. Diskretna analogija Dirakove funkcije je Kroneker delta funkcija, koja je obično definisana u konačnom domenu i uzima vrednosti između 0 i 1.

Iako gledano iz čisto matematičke strane, Dirakova delta funkcija nije striktna funkcija, odnosno nije funkcija u pravom smislu tih reči. Integral bilo koje realne funkcije koja doseže do beskonačnosti i ima vrednost u svim tačkama 0, a samo u jednoj tački vrednost 1 imao bi vrednost 0, a ne 1 što je slučaj sa δ funkcijom. Dirakova delta funkcija ima smisao jedino kada se pojavljuje kao matematički objekat unutar integrala. Formalno se mora definisati kao distribucija ili mera. U mnogim primenama, δ funkcija predstavlja graničnu vrednost niza funkcija normalnih distribucija sa tačkom nagomilavanja u nuli, iako su približne vrednosti ovih raspodela samo približna vrednost δ funkcije.

Definicija

Dirakova delta funkcija je najpribližnije rečeno funkcija na realnoj pravoj čija je vrednost svugde nula, osim u koordinatnom početku. gde je njena vrednost beskonačna,

δ ( x ) = { + ∞ , x = 0 0 , x ≠ 0 {\displaystyle \delta (x)={\begin{cases}+\infty ,&x=0\\0,&x\neq 0\end{cases}}}

i defisana da zadovoljava identitet da je njen integral u intervalu od − ∞ {\displaystyle -\infty } do + ∞ {\displaystyle +\infty } jednak 1,

∫ − ∞ ∞ δ ( x ) d x = 1. {\displaystyle \int _{-\infty }^{\infty }\delta (x)\,dx=1.}

δ funkcija se formalno definiše kao distribucija ili mera.

Sličnost sa Kronekerovom delta funkcijom

Kronekerova delta funkcija se za cele brojeve i i j definiše kao:

δ i j = { 1 i = j 0 i ≠ j . {\displaystyle \delta _{ij}={\begin{cases}1&i=j\\0&i\not =j.\end{cases}}}

Tada za sve nizove ( a i ) i ∈ Z {\displaystyle (a_{i})_{i\in \mathbf {Z} }} koji su beskonačni u oba pravca (dosežu i do + ∞ {\displaystyle +\infty } i do − ∞ {\displaystyle -\infty } ), važi:

∑ i = − ∞ ∞ a i δ i k = a k . {\displaystyle \sum _{i=-\infty }^{\infty }a_{i}\delta _{ik}=a_{k}.}

Slično, za bilo koju realnu ili kompleksnu funkciju ƒ neprekidnu u R, Dirakova delta funkcija zadovoljava osobinu:

∫ − ∞ ∞ f ( x ) δ ( x − x 0 ) d x = f ( x 0 ) . {\displaystyle \int _{-\infty }^{\infty }f(x)\delta (x-x_{0})\,dx=f(x_{0}).}

Povezanost osobina ovih dveju funkcija čini Kronekerovu delta funkciju diskretnom analogijom Dirakove delta funkcije na skupu [ 0 , 1 ] {\displaystyle [0,1]} .

Primena

δ funkcija u fizici predstavlja idealizovani centar mase. Dirakova delta distribucija se koristi u teoriji verovatnoće za diskretnu raspodelu. Diskretizovana δ funkcija je ključna za formulisanje ortonormalnosti u kvantnoj mehanici. Koristi se i u teoriji konstrukcija za opisivanje prolaznog opterećenja ili tačke opterećenja u strukturama.

Vidi još

Literatura

  • Aratyn, Henrik; Rasinariu, Constantin (2006). A short course in mathematical methods with Maple. World Scientific. ISBN 981-256-461-6. .
  • Arfken, G. B.; Weber, H. J. (2000). Mathematical Methods for Physicists (5th изд.). Boston, MA: Academic Press. ISBN 978-0-12-059825-0. .
  • Bracewell, R. (1986), The Fourier Transform and Its Applications (2nd изд.), McGraw-Hill .
  • Córdoba, A., „La formule sommatoire de Poisson”, C.R. Acad. Sci. Paris, Series I, 306: 373—376 .
  • Courant, Richard; Hilbert, David (1962), Methods of Mathematical Physics, Volume II, Wiley-Interscience .
  • Davis, Howard Ted; Thomson, Kendall T (2000). Linear algebra and linear operators in engineering with applications in Mathematica. Academic Press. ISBN 0-12-206349-X. 
  • Dieudonné, Jean (1976). Treatise on analysis. Vol. II. New York: Academic Press [Harcourt Brace Jovanovich Publishers]. ISBN 978-0-12-215502-4. MR 0530406. .
  • Dieudonné, Jean (1972), Treatise on analysis. Vol. III, Boston, MA: Academic Press, MR 0350769 
  • Dirac, Paul (1958). Principles of quantum mechanics (4th изд.). Oxford at the Clarendon Press. ISBN 978-0-19-852011-5. .
  • Driggers, Ronald G. (2003). Encyclopedia of Optical Engineering. CRC Press. ISBN 978-0-8247-0940-2. .
  • Federer, Herbert (1969). Geometric measure theory. Die Grundlehren der mathematischen Wissenschaften. 153. New York: Springer-Verlag. стр. xiv+676. ISBN 978-3-540-60656-7. MR 0257325. .
  • Gel'fand, I.M.; Shilov, G.E. (1966—1968), Generalized functions, 1—5, Academic Press .
  • Hartman, William M. (1997). Signals, sound, and sensation. Springer. ISBN 978-1-56396-283-7. .
  • Hewitt, E; Stromberg, K (1963), Real and abstract analysis, Springer-Verlag .
  • Hörmander, L. (1983). The analysis of linear partial differential operators I. Grundl. Math. Wissenschaft. 256. Springer. ISBN 3-540-12104-8. MR 0717035. .
  • Isham, C. J. (1995). Lectures on quantum theory: mathematical and structural foundations. Imperial College Press. ISBN 978-81-7764-190-5. .
  • John, Fritz (1955), Plane waves and spherical means applied to partial differential equations, Interscience Publishers, New York-London, MR 0075429 .
  • Lang, Serge (1997). Undergraduate analysis. Undergraduate Texts in Mathematics (2nd изд.). Berlin, New York: Springer-Verlag. ISBN 978-0-387-94841-6. MR 1476913. .
  • Laugwitz, D. (1989), „Definite values of infinite sums: aspects of the foundations of infinitesimal analysis around 1820”, Arch. Hist. Exact Sci., 39 (3): 195—245, doi:10.1007/BF00329867 .
  • Levin, Frank S. (2002). „Coordinate-space wave functions and completeness”. An introduction to quantum theory. Cambridge University Press. стр. 109ff. ISBN 0-521-59841-9. 
  • Li, Y. T.; Wong, R. (2008), „Integral and series representations of the Dirac delta function”, Commun. Pure Appl. Anal., 7 (2): 229—247, MR 2373214, doi:10.3934/cpaa.2008.7.229 .
  • de la Madrid, R.; Bohm, A.; Gadella, M. (2002), „Rigged Hilbert Space Treatment of Continuous Spectrum”, Fortschr. Phys., 50 (2): 185—216, Bibcode:2002ForPh..50..185D, arXiv:quant-ph/0109154 Слободан приступ, doi:10.1002/1521-3978(200203)50:2<185::AID-PROP185>3.0.CO;2-S .
  • McMahon, D. (2005). „An Introduction to State Space”. Quantum Mechanics Demystified, A Self-Teaching Guide. Demystified Series. New York: McGraw-Hill. стр. 108. ISBN 0-07-145546-9. doi:10.1036/0071455469. Архивирано из оригинала 26. 03. 2016. г. Приступљено 17. 3. 2008. .
  • van der Pol, Balth.; Bremmer, H. (1987). Operational calculus (3rd изд.). New York: Chelsea Publishing Co. ISBN 978-0-8284-0327-6. MR 904873. .
  • Rudin, W. (1991). Functional Analysis (2nd изд.). McGraw-Hill. ISBN 0-07-054236-8. .
  • Soares, Manuel; Vallée, Olivier (2004), Airy functions and applications to physics, London: Imperial College Press .
  • Saichev, A I; Woyczyński, Wojbor Andrzej (1997). „Chapter1: Basic definitions and operations”. Distributions in the Physical and Engineering Sciences: Distributional and fractal calculus, integral transforms, and wavelets. Birkhäuser. ISBN 0-8176-3924-1. 
  • Schwartz, L. (1950), Théorie des distributions, 1, Hermann .
  • Schwartz, L. (1951), Théorie des distributions, 2, Hermann .
  • Stein, Elias; Weiss, Guido (1971). Introduction to Fourier Analysis on Euclidean Spaces. Princeton University Press. ISBN 0-691-08078-X. .
  • Strichartz, R. (1994). A Guide to Distribution Theory and Fourier Transforms. CRC Press. ISBN 0-8493-8273-4. .
  • Vladimirov, V. S. (1971). Equations of mathematical physics. Marcel Dekker. ISBN 0-8247-1713-9. .
  • Yamashita, H. (2006), „Pointwise analysis of scalar fields: A nonstandard approach”, Journal of Mathematical Physics, 47 (9): 092301, Bibcode:2006JMP....47i2301Y, doi:10.1063/1.2339017 
  • Yamashita, H. (2007), „Comment on "Pointwise analysis of scalar fields: A nonstandard approach" [J. Math. Phys. 47, 092301 (2006)]”, Journal of Mathematical Physics, 48 (8): 084101, Bibcode:2007JMP....48h4101Y, doi:10.1063/1.2771422 

Spoljašnje veze

  • Hazewinkel Michiel, ур. (2001). „Delta-function”. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104. 
  • KhanAcademy.org video lesson
  • The Dirac Delta function, a tutorial on the Dirac delta function.